Evaluate The Infinite Geometric Series

Infinite Geometric Series Formula

Earlier learning the space geometric series formula, permit us recall what is a geometric series. A geometric series is the sum of a sequence wherein every successive term contains a constant ratio to its preceding term. An Infinite geometric serial has an infinite number of terms and tin can be represented equally a, ar, ar², ..., to ∞. Allow united states learn the infinite geometric serial formula in the upcoming section.

What Is Infinite Geometric Series Formula?

The sum of the infinite geometric series formula of the space series formula is also known as the sum of infinite GP. The infinite series formula if the value of r is such that −1<r<i, tin be given as,

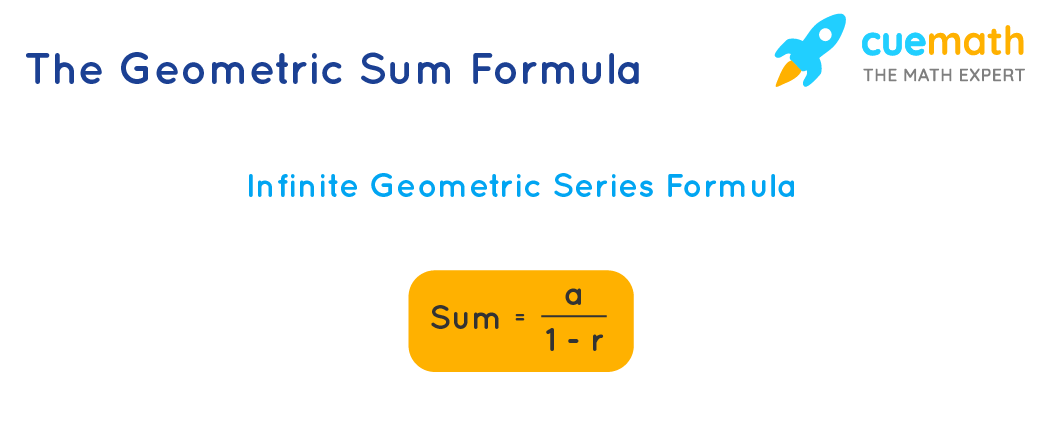
Sum = a/(1-r)

Where,

a = first term of the series
r = common ratio between two consecutive terms and −ane < r < ane

Infinite Geometric Series Formula

Infinite Geometric Series formula

The geometric series converges to a sum only if r < one. If r> 1, the serial does not converge and doesn't have a sum. For example 8, 12, 18, 27, .... is the given geometric serial.

To find the sum : 8 + 12 + 18 + 27 + ..... , we notice that a = eight and r = 12/8 = 18/ 12 = 3/two

Here r > 1. Thus the sum does not converge and the serial has no sum.

Have questions on basic mathematical concepts?

Become a problem-solving champ using logic, not rules. Learn the why behind math with our certified experts

Volume a Free Trial Class

Let u.s.a. at present have a look at a few solved examples using the Space Series Formula.

Case i: Find the sum of the terms 1/9 + 1/27 + one/81 + ... to ∞?

Solution: To find: Sum of the geometric serial

Given:

a = 1/9, r = i/3

Using the infinite geometric series formula,

Due south_northward = a /(1-r)

S_n = (ane/9)( 1 - one/3)

South_n = 1/6

Answer: The sum of the given terms is i/six

Case 2: Calculate the sum of series ane/5, 1/x, i/20, .... if the series contains infinite terms.

Solution: To find: Sum of the geometric series

Given:

a = 1/v, r = i/5

Using the space geometric series formula,

Sum = a /(1-r)

= (one/5)( 1 - i/5)

= ane/four

Answer: The sum of the given terms is 1/four

Example three: Find the sum of the geometric series 125, 25, 5, 1......∞

Solution: The series is,125+25+v+ane+ ........ a =125, and = 25/125 = ane/5

Using the space geometric series formula,

Sum = a /(1-r)
= 125/(one-1/v)

= 125/(4/5)

= 625/4

Thus sum to infinity terms is

Answer: Thus sum to infinity in the given series 125+25+5+1+ ........ =& 625/4

FAQs on Geometric Infinite Series Formula

What Is Geometric Infinite Series Formula?

The infinite geometric series formula is used to observe the sum of all the terms in the geometric serial without actually calculating them individually. The space geometric series formula is given as:

\(S_{northward}=\dfrac{a}{i-r}\)

Where

a is the first term
r is the mutual ratio

A tangent of a circle in geometry is defined equally a straight line that touches the circle at just 1 point.

Can the Sum of an Infinite Geometric Serial be Negative?

The sum of an infinite series implies that the series is geometric and an infinite arithmetic serial can never converge. And then if the common ratio is positive in that location can be no negative sum.

What Is the Sum of Infinite GP?

The sum to infinite GP means, the sum of terms in an infinite GP. The infinite geometric series formula is S∞ = a/(1 – r), where a is the first term and r is the mutual ratio.

What Is a and r in Space Series Formula?

In finding the sum of the given space geometric series If r<1 is then sum is given as Sum = a/(1-r). In this infinite series formula, a = first term of the serial and r = common ratio between ii consecutive terms and −1<r<1